connecting the inverting and non-inverting input terminals of the
op-amp as shown in figure 6.31
When calculating the output impedance we still assumed an infinite
input impedance.
In this section we will calculate the finite input impedance assuming
a zero output impedance.
We consider a model that assumes an internal resistor
connecting the inverting and non-inverting input terminals of the
op-amp as shown in figure 6.31
Consider an inverting amplifier and remove the input resistor so that the input impedance can be calculated directly at the amplifier's input terminals.
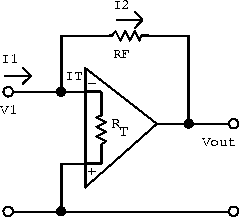
Figure 6.31: Model for calculating the input impedance of
the inverting amplifier.
The input impedance is defined by
and the current at the summing junction is
The current through the feedback resistor is
and the output voltage is related to 
by the open-loop gain
The resulting input impedance is thus
For large A
The closed-loop input impedance is thus small and almost independent of
the large of the operational amplifier.
Now consider the non-inverting amplifier shown in figure 6.32.
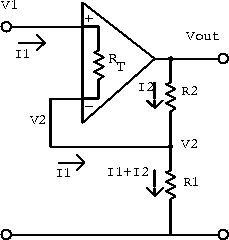
Figure 6.32: Model for calculating the input impedance of
the non-inverting amplifier.
The student should calculate the input impedance by recognizing that
is much less than 
, since is much greater
than 
or 
.
Your result should be
where 
is the closed-loop gain of the amplifier.
Notice that in contrast to the low input impedance for the inverting
amplifier, the non-inverting amplifier exhibits a closed-loop input
impedance that is much larger than the open-loop value .
Narippawaj Ngernvijit